Square cupola
| Square cupola | |
|---|---|
| Type | Johnson J3 - J4 - J5 |
| Faces | 4 triangles 1+4 squares 1 octagon |
| Edges | 20 |
| Vertices | 12 |
| Vertex configuration | 8(3.4.8) 4(3.43) |
| Symmetry group | C4v |
| Dual polyhedron | - |
| Properties | convex |
| Net | |
In geometry, the square cupola, sometimes called lesser dome, is one of the Johnson solids (J4). It can be obtained as a slice of the rhombicuboctahedron. As in all cupolae, the base polygon has twice as many edges and vertices as the top; in this case the base polygon is an octagon.
Contents |
Formulae
The following formulae for volume, surface area, and circumradius can be used if all faces are regular, with edge length a:[1]
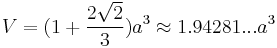
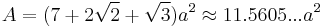
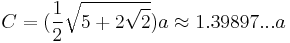
Dual polyhedron
The dual of the square cupola has 16 triangular faces:
| Dual square cupolaamid | Net of dual |
|---|---|
References
- ^ Stephen Wolfram, "Square cupola" from Wolfram Alpha. Retrieved July 20, 2010.